The following three pictures are representations of the total sky.

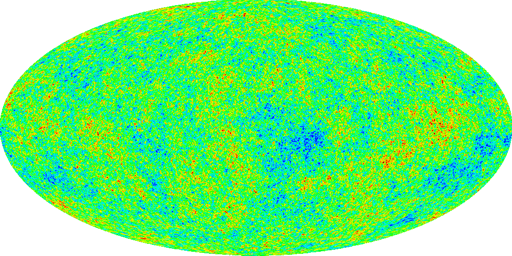
• Picture 1 shows the Planck CMB radiation observation of 21 March 2013
• Picture 2 shows a CMB radiation simulation of 21 March 2013 with large and small bubbles.
• Picture 3 shows a CMB radiation simulation with only small bubbles.

Planck Scale 1 to 1 Temperature -514 to 517 Picture 1

Planck Simulation with large and small bubbles Temperature -815 to 679 Picture 2

Planck Simulation with small bubbles Temperature -904 to 684 Picture 3

• Picture #1 Average temperature = -44.10 spread = 148.03
• Picture #2 Average temperature = -42.37 Spread = 148.44
• Picture #3 Average temperature = -44.27 Spread = 130.38

The simulation of Picture #2 is performed by placing small temperature hills and valleys at random places on the surface of a sphere. This are the so called bubbles. The hills and valleys are placed in pairs. The size of each pair is the same. For a hill the temperature values are positif. For the paired valley the temperature values are the same but negatif. By doing this the average temperature value is kept (almost) constant. The initial average temperature is -44.
The simulation is performed by two types of hills and valleys (bubbles): large one's and small one's.

• The large one have an average radius of 30 pixels (small l values)
• The small one's have a radius of 1 pixel (large l values)

The total number of large one's (hills, valleys, bubbles) is 100000. The total number of small one's is 1000000.
The radius of the sphere of the simulation is 256 pixels. The radius of Picture #1,#2 and #3 is 128 pixels.

When you compare the picture #1 with picture #2 they are rather identical. The most important difference that Picture #2 does not contain any information about the physical state of the Universe.
Picture #3 shows only the small hills and valleys. This picture is important because in order to calculate the cosmological parameters only the large l values are used. See also . Reflection part 2

To observe the Power Spectrum based on Plank data, the Bubble Universe and WMAP data accordingly to the methode described at page 242 in the book "The Inflationary Universe" select this link: Critical evaluation of Power Spectrum calculation in the book "The Inflationary Universe" by Alan H. Guth

Reflection part 1

Document: Cosmological-Parameter Determination with Microwave Background Maps by G Jungman (23 May 1996) starts with the following sentence:

The angular power spectrum of the cosmic microwave background (CMB) contains information on virtually all cosmological parameters of interest, including the geometry of the Universe (Omega), the baryon density, the Hubble constant (h), the cosmological constant (Lambda), the number of light neutrinos, the ionization history, and the amplitudes and spectral indices of the primordial scalar and tensor perturbation spectra.

This is a rather astonishing sentence because the sentence only reflects the situation of Picture 1 but not of Picture 2.
The cosmic microwave background radiation (the temperature profile) can be described as a superposition of a dipole (l=2) a quadrupole (l=3) a octopole (l=4) and all types of multipoles until l=300 and higher. For difficulties involved to calulate the multipoles select: Low-order multipole maps of cosmic microwave background anisotropy derived from WMAP by P. Bielewicz K.M. Gorski and A.J. Banday (April 2004)

The problem is that electrical multipoles have a rather strict mathematical definition.

For example select The Static Electric Dipole, Quadrupole, & Multipoles by Professor K.E. Oughstun what is involved.

To get an idea about magnetic multipoles select:

Analytical Formulae For Magnetic Multipoles by M. Basetti and C. Biscari

The issue is that it is rather easy to calculate a field starting from a certain distribution, but very difficult to calculate the physical distribution (the source) starting from a distribution. That is what you want to do. You start from the cosmic micro wave background radiation and you want to calculate the multipoles that caused this radiation. What is more difficult the background radiation is a measurement on a sphere while the real cause is what happening in the inside and what is hidden. The remark can be made that what you try to do ie. starting from a sphere, has any physical bearing

To investigate this whole process in more detail consider the next:

• For l = 2 (dipole) you have one hill and one valley (hot and cold) spot on the surface of the sphere. To describe the hill you need one point and the strength. The position of the valley is than "fixed". In total 3 parameters.
• For l = 3 (quadrupole) there are two hills and two valleys. The physical shape includes 4 faces, 4 vertices and 6 edges of all equal sizes (ribs). In this case you need the same three parameters and one more parameter to reflect rotation. The position of the two valleys is than fixed. In total four parameters.
• For l = 5 there are four hills and four valleys. The physical shape represents a cube. 8 faces, 8 vertices and 12 edges of all equal sizes (ribs). Also here you need four parameters.
• For l = 100 there are 101 hills and 101 valleys. You need at least four parameters.

To calculate all these multipoles I expect is a very complicated task.

More to read:

• A high resolution foreground cleaned CMB map from WMAP by Max Tegmark (Penn), Angelica de Oliveira-Costa (Penn), Andrew Hamilton (JILA) 26 july 2003
  Fig 15 shows three images: Quadrupole, Octopole, and hexadecapole.
• Quadrupole–octopole alignment of CMB related to primordial power spectrum with dipolar modulation in anisotropic spacetime Zhe Chang, Xin Li, Sai Wang 23 Dec 2013
  Technical document explains what is involved.
• Planck 2013 results. XXIII. Isotropy and statistics of the CMB
  

  This document at page 20 in Figure shows two images

  • One of the derived temperature quadrupole (two blue and two brown "circles")
  • and one of the derived temperature octopole (three blue and three brown circles) of both 35 micro Kelvin.

  Those two images are interesting because they seem to match with the anisotropies of the CMB radiation. In fact all the high angle multiplepoles seem to be important inorder to explain structure in the CMB radiation.
  At page 43 we can read:

  It is well-known that the quadrupole and octopole have low amplitudes relative to the best-fit cosmological power-spectrum. The contribution of those multipoles to cosmological parameter estimation is very small etc
  Moreover, this conclusion is a consequence of the fact that the cosmological parameters are strongly influenced by the l = 1000–1500 range, previously inaccessible to WMAP.

• Planck 2013 results. XV. CMB power spectra and likelihood
  
  The resulting spectrum is shown in Fig. 37 together with the corresponding best-fit six-parameter Lambda CDM model.
  Planck 2013 results. XVI. Cosmological parameters
  

  At page 7 (2.1.5 Power spectra) we read:

  Our baseline numerical Boltzmann code is camb (March 2013) a parallelized line-of-sight code developed from cmbfast and Cosmics which calculates the lensed CMB temperature and polarization power spectra.

  At page 47 (6.4. Big bang nucleosynthesis) we read:

  We also assume that there is no significant entropy increase between BBN and the present day, so that our CMB constraints on the baryon-to-photon ratio can be used to compute primordial abundances.

The following three documents challenge the Planck results

• Simple but challenging: the Universe according to Planck 21 March 2013
  

  This document at the end shows a clear image of the cold spot.
  In this document we can read:

  If the fabric of the cosmos is not isotropic on scales so large that extend beyond the horizon of the 'patch' of the Universe that we can access with observations, its global geometry would be rather complex: this could force bundles of light rays into highly intricate paths where they would be significantly focussed. As CMB photons have travelled across the Universe for most of its history, they might have experienced this effect, resulting in the anomalous pattern of the CMB observed across the sky.

  The part of the universe we can access with observations is very small. The assumption that the universe is not uniform is more realistic than that the universe is homogene and uniform.
  The CMB photons, we observe, are supposed to be an undisturbed and clear image of what happened 300000 years after the Big Ban. That means that the photons travelled in a straight path towards us after being created 17 billion years ago. This seems a rather simple picture. In reality to assume that the path is much more complex seems to be a much more realistic assumption. At the same time this raises also the question to what extend the CMB radiation gives as reliable information about the total universe enlarge.

• New planck data challenges our understanding of the universe This document reads:

  One way to explain the anomalies is to propose that the Universe is in fact not the same in all directions on a larger scale than we can observe. In this scenario, the light rays from the CMB may have taken a more complicated route through the Universe than previously understood, resulting in some of the unusual patterns observed today.

  The fact that photons don't follow straight lines is easy to accept because we know that there is micro lensing. The reverse is more difficult to accept. The consequences are severe: because this indicates that the physical interpretation of the CMB radiation (as what they mean) is much less secure than what we currently agree upon.

• Inflationary paradigm in trouble after Planck2013

Reflection part 2

The interpretation of the first peak in the CMB radiation is that the universe is flat.

See also The Mathematical Universe by Max Tegmark - Reflection part 3 - flat universe
See also Wikipedia CMB Primary anisotropy

The chalenge is to calculate the Power Spectrum of Picture #3 and to see what and where the peaks are. Because Picture #3 is based on hills and valleys of one pixel I expect that there will be only one peak. The radius of the sphere is 256 pixels.
The same exercise should be done for Picture #2.

For more about the CMB radiation read this:

• WMAP 2009 comparison versus Planck 21 march 2013
  
• Friedmann's equation - Question 13